I've just finished a five week stint running our school's Y7 and Y8 STEM Club - and what fun it's been! I chose the theme of 'fractallations' - a fractal-tessellation hybrid that would allow me to include lots of my favourite things, including, you guessed it, some paper folding :)
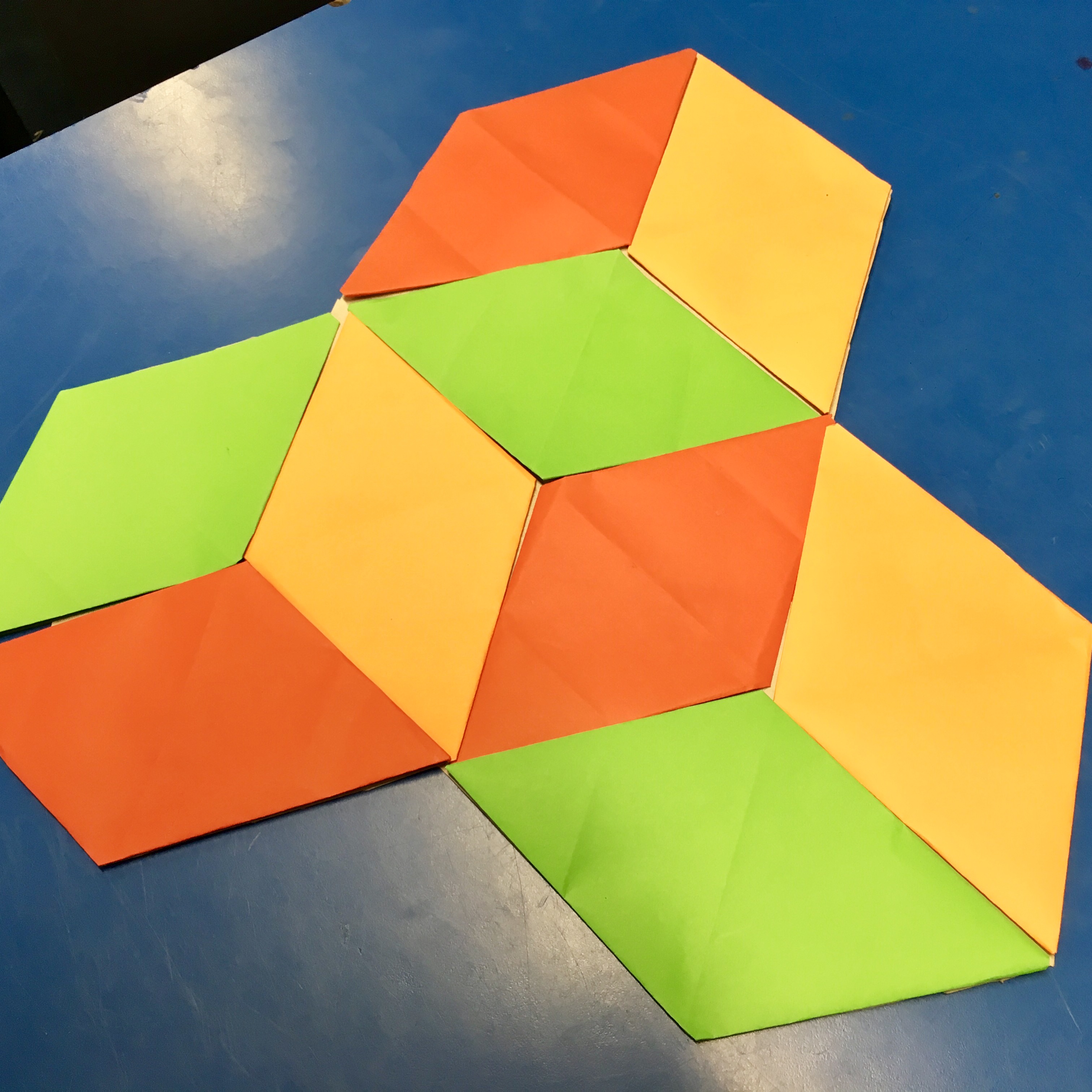
We started by exploring what characterises a 'fractal' pattern (in visual terms) and then investigated and drew Sierpinski Triangles using this excellent resource from the STEM Learning centre. Then, in week 2, we looked at tessellations, and learnt how to fold paper polygons with which to create some tessellations of our own (below). For this I used my 'Patchwork Paper Patterns' presentation containing Liz Meenan's instructions - available on the Mathematical Art Lessons page.
With the basics now in hand, we could start folding Christmas fractals...
Koch Snowflakes
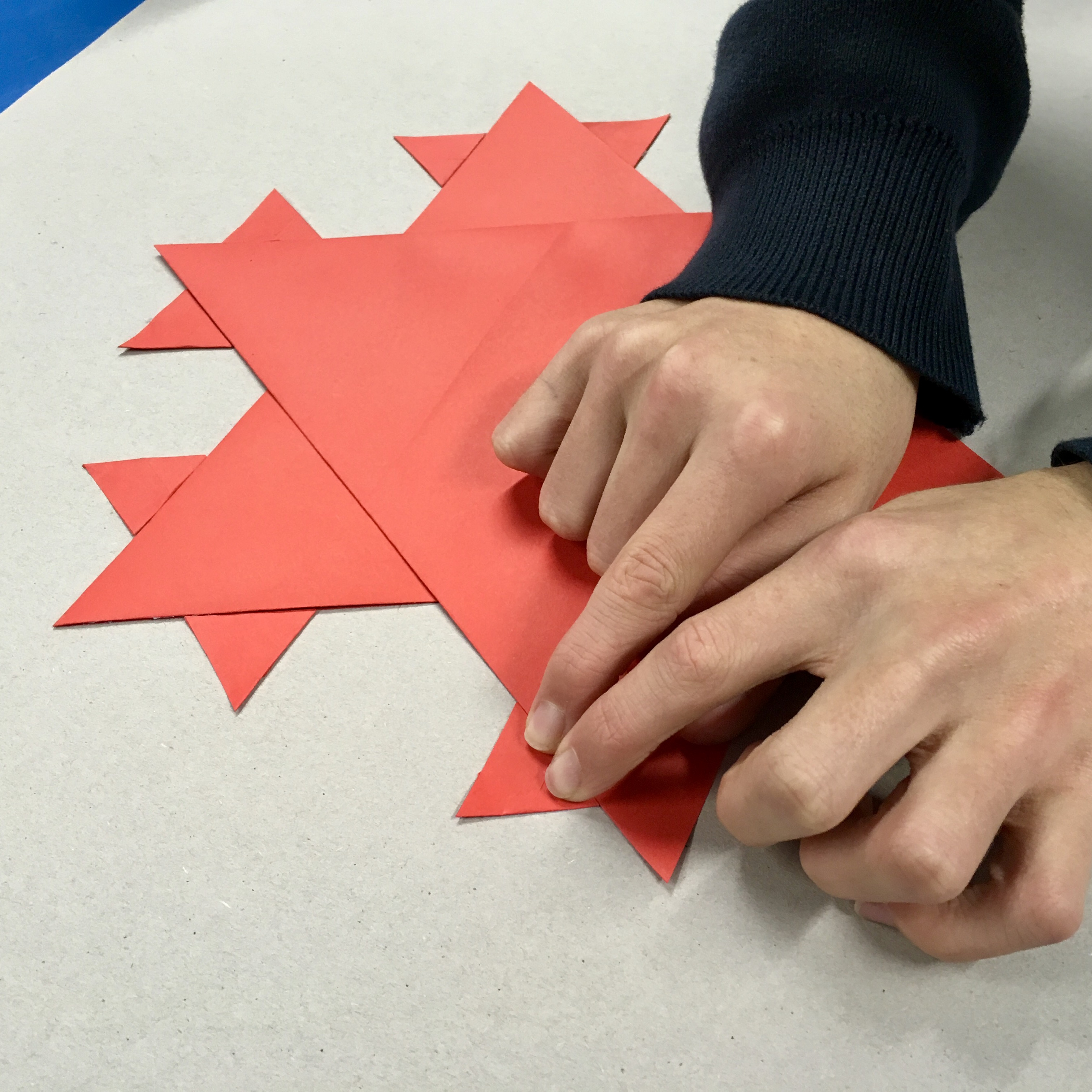
Inspiration for this activity struck whilst I was attending the recent ATM/MA 'Problem Solving with Paper Folding' course delivered by Fran Watson of @nrichmaths. We were introduced to a very clever method for folding an equilateral triangle from A4 paper that I'd not come across before. I'd been wanting to make Koch Snowflakes with the students, and now I had my medium!
|
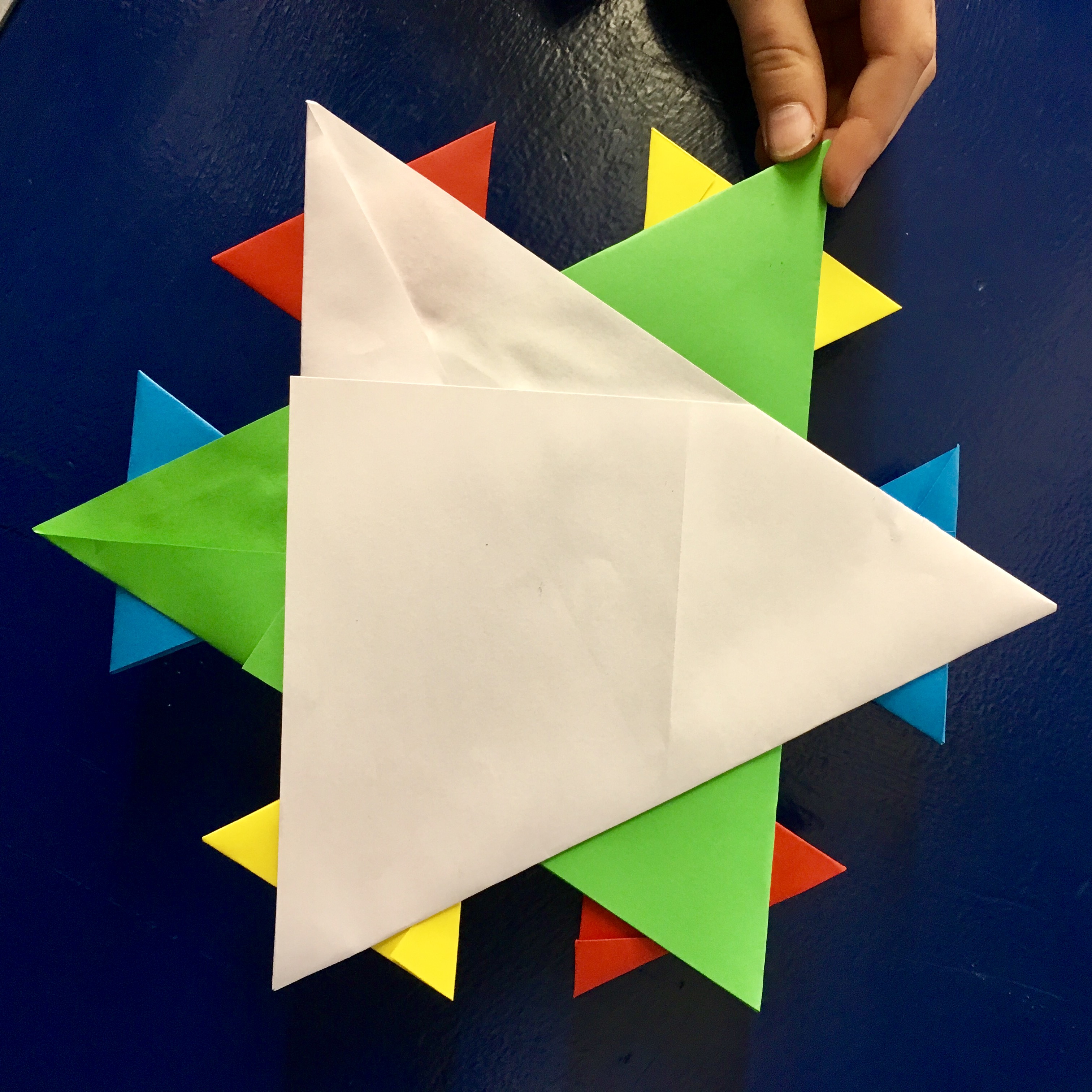
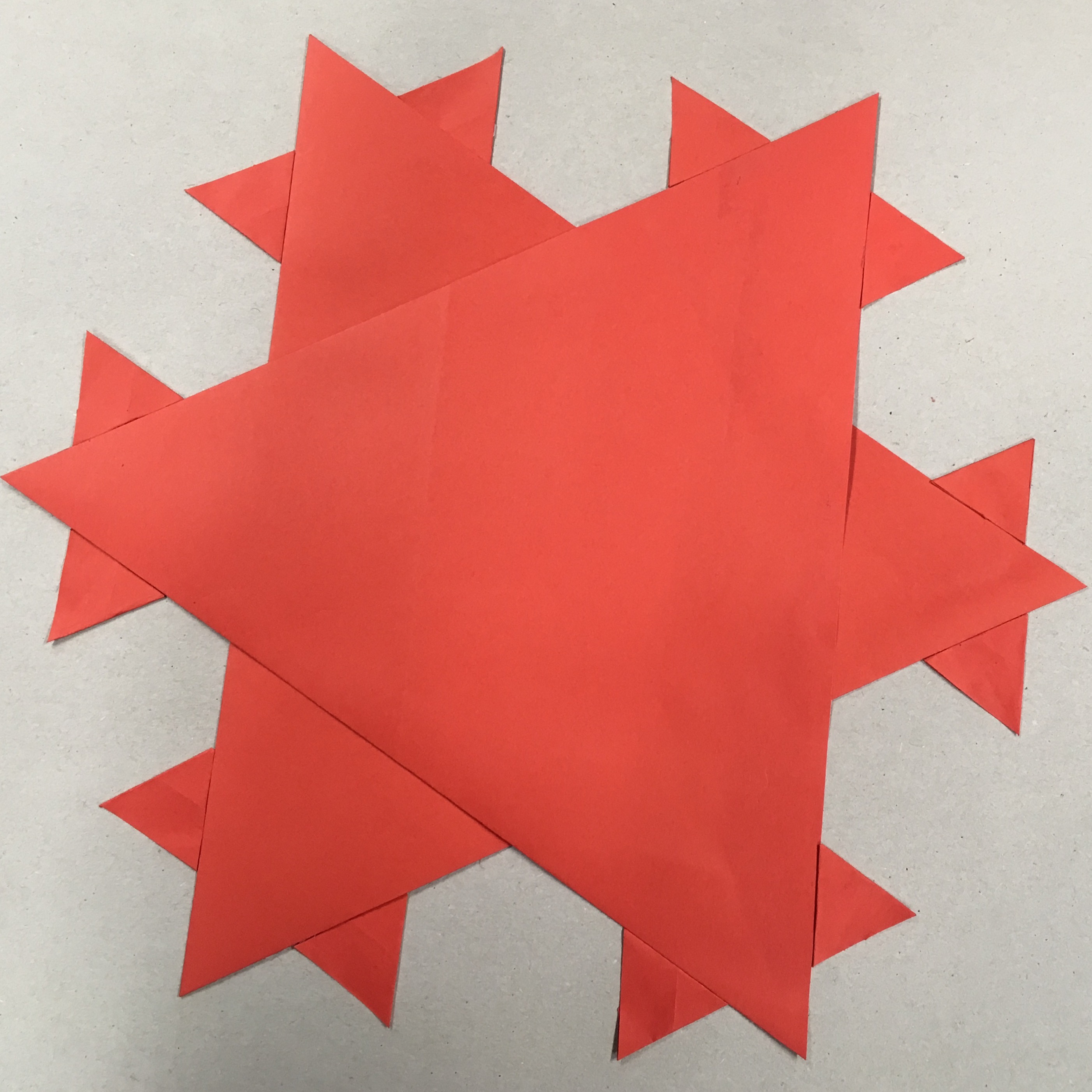
After treating the students to a 'fractal zoom' (loads available on Youtube) we looked at the Koch Snowflake in more detail, discussing its structure and how it 'grew'. I then projected the instructions for folding the equilateral triangle (I used this resource from Arbelos; alternatively this excellent @MEImaths presentation shows it step-by-step) and let the students figure it out by themselves. Once they'd nailed the fold, I set them the challenge of using different A-sized paper to create their own fractal snowflakes. They did really well!
|
However, things got very small, very quickly. Next time I might suggest they start with a much larger central triangle tessellated from four, or even nine, A4-sized ones, and make GIANT fractal snowflakes!
Reflecting on this activity afterwards it struck me that it had potential to form the basis of a length and area scale factors investigation. In addition, older students could be set the challenge of calculating the (finite) overall area of the snowflake.
Fractal Christmas Tree
No mathematical Christmas can be considered complete without Matt Parker's (@standupmaths) ThinkMaths' Fractal Christmas tree! This fab resource, available here, is a great test of teamwork and problem solving skills, and I was so impressed with how the students tackled it. Deciding to call themselves 'Christmas engineers', they organised themselves into teams, set up production lines and got completely stuck into the challenge. No pics of their tree yet, but this is one I made with students last year.
Fractal Christmas Cards
We didn't have time to make these during STEM Club in the end, so I'm planning to make them with my Y12 further mathematicians after their end of term assessment instead (they are a very spoilt bunch). Full instructions and resources are available on the Fractal Foundation website here. Alternatively Emma Morgan (@em0rgan) has put together this useful walk-through video.
Her students' cards look fantastic!
|
Happy festive fractal folding :)






 RSS Feed
RSS Feed

